Truncated pentakis dodecahedron

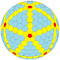
The truncated pentakis dodecahedron is a convex polyhedron constructed as a truncation of the pentakis dodecahedron. It is Goldberg polyhedron G_V(3,0), with pentagonal faces separated by an edge-direct distance of 3 steps.

Truncated pentakis dodecahedron
Conway notation	tkD
Goldberg polyhedron	GPV(3,0) or {5+,3}3,0
Fullerene	C180[1]
Faces	92: 12 pentagons 20+60 hexagons
Edges	270 (2 types)
Vertices	180 (2 types)
Vertex configuration	(60) 5.6.6 (120) 6.6.6
Symmetry group	Icosahedral (Ih)
Dual polyhedron	Hexapentakis truncated icosahedron
Properties	convex

Related polyhedra

It is in an infinite sequence of Goldberg polyhedra:

Index	GP(1,0)	GP(2,0)	GP(3,0)	GP(4,0)	GP(5,0)	GP(6,0)	GP(7,0)	GP(8,0)...
Image	D	kD	tkD
Duals	I	cD	ktI

See also

• Near-miss Johnson solid
• Truncated tetrakis cube