List of spherical symmetry groups
From Wikipedia, the free encyclopedia
Finite spherical symmetry groups are also called point groups in three dimensions. There are five fundamental symmetry classes which have triangular fundamental domains: dihedral, cyclic, tetrahedral, octahedral, and icosahedral symmetry.
 Involutional symmetry Cs, (*) [ ] = |
 Cyclic symmetry Cnv, (*nn) [n] = |
 Dihedral symmetry Dnh, (*n22) [n,2] = | |
| Polyhedral group, [n,3], (*n32) | |||
|---|---|---|---|
 Tetrahedral symmetry Td, (*332) [3,3] = |
 Octahedral symmetry Oh, (*432) [4,3] = |
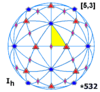 Icosahedral symmetry Ih, (*532) [5,3] = | |
This article lists the groups by Schoenflies notation, Coxeter notation,[1] orbifold notation,[2] and order. John Conway uses a variation of the Schoenflies notation, based on the groups' quaternion algebraic structure, labeled by one or two upper case letters, and whole number subscripts. The group order is defined as the subscript, unless the order is doubled for symbols with a plus or minus, "±", prefix, which implies a central inversion.[3]
Hermann–Mauguin notation (International notation) is also given. The crystallography groups, 32 in total, are a subset with element orders 2, 3, 4 and 6.[4]