Maximum and minimum
Largest and smallest value taken by a function takes at a given point / From Wikipedia, the free encyclopedia
Dear Wikiwand AI, let's keep it short by simply answering these key questions:
Can you list the top facts and stats about Maxima and minima?
Summarize this article for a 10 years old
In mathematical analysis, the maximum (PL: maxima or maximums) and minimum (PL: minima or minimums) of a function, known generically as extremum (PL: extrema), are the largest and smallest value of the function, either within a given range (the local or relative extrema), or on the entire domain (the global or absolute extrema).[1][2][3] Pierre de Fermat was one of the first mathematicians to propose a general technique, adequality, for finding the maxima and minima of functions.
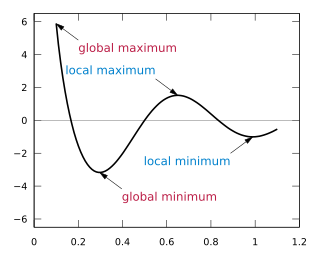
As defined in set theory, the maximum and minimum of a set are the greatest and least elements in the set, respectively. Unbounded infinite sets, such as the set of real numbers, have no minimum or maximum.