Hemi-dodecahedron

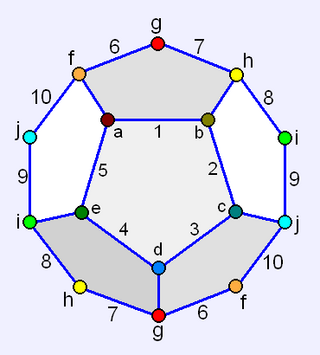
In geometry, a hemi-dodecahedron is an abstract, regular polyhedron, containing half the faces of a regular dodecahedron. It can be realized as a projective polyhedron (a tessellation of the real projective plane by 6 pentagons), which can be visualized by constructing the projective plane as a hemisphere where opposite points along the boundary are connected and dividing the hemisphere into three equal parts.

Hemi-dodecahedron
Decagonal Schlegel diagram
Type	Abstract regular polyhedron Globally projective polyhedron
Faces	6 pentagons
Edges	15
Vertices	10
Euler char.	χ = 1
Vertex configuration	5.5.5
Schläfli symbol	{5,3}/2 or {5,3}5
Symmetry group	A5, order 60
Dual polyhedron	hemi-icosahedron
Properties	Non-orientable

It has 6 pentagonal faces, 15 edges, and 10 vertices.

Projections

It can be projected symmetrically inside of a 10-sided or 12-sided perimeter:

Petersen graph

From the point of view of graph theory this is an embedding of the Petersen graph on a real projective plane. With this embedding, the dual graph is K₆ (the complete graph with 6 vertices) --- see hemi-icosahedron.

The six faces of the hemi-dodecahedron depicted as colored cycles in the Petersen graph

See also

• 57-cell – an abstract regular 4-polytope constructed from 57 hemi-dodecahedra.
• hemi-icosahedron
• hemi-cube
• hemi-octahedron

References

• McMullen, Peter; Schulte, Egon (December 2002), "6C. Projective Regular Polytopes", Abstract Regular Polytopes (1st ed.), Cambridge University Press, pp. 162–165, ISBN 0-521-81496-0

External links

• The hemidodecahedron