Quadrifolium

The quadrifolium (also known as four-leaved clover^[1]) is a type of rose curve with an angular frequency of 2. It has the polar equation:

${\displaystyle r=a\cos(2\theta ),\,}$

Rotated quadrifolium

Quadrifolium created with gears

This article is about the geometric shape. For the plant, see Four-leaf clover. For the symmetrical shape framework, see Quatrefoil.

with corresponding algebraic equation

${\displaystyle (x^{2}+y^{2})^{3}=a^{2}(x^{2}-y^{2})^{2}.\,}$

Rotated counter-clockwise by 45°, this becomes

${\displaystyle r=a\sin(2\theta )\,}$

with corresponding algebraic equation

${\displaystyle (x^{2}+y^{2})^{3}=4a^{2}x^{2}y^{2}.\,}$

In either form, it is a plane algebraic curve of genus zero.

The dual curve to the quadrifolium is

${\displaystyle (x^{2}-y^{2})^{4}+837(x^{2}+y^{2})^{2}+108x^{2}y^{2}=16(x^{2}+7y^{2})(y^{2}+7x^{2})(x^{2}+y^{2})+729(x^{2}+y^{2}).\,}$

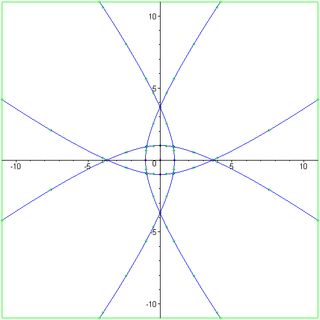Dual quadrifolium

The area inside the quadrifolium is ${\displaystyle {\tfrac {1}{2}}\pi a^{2}}$, which is exactly half of the area of the circumcircle of the quadrifolium. The perimeter of the quadrifolium is

${\displaystyle 8a\operatorname {E} \left({\frac {\sqrt {3}}{2}}\right)=4\pi a\left({\frac {(52{\sqrt {3}}-90)\operatorname {M} '(1,7-4{\sqrt {3}})}{\operatorname {M} ^{2}(1,7-4{\sqrt {3}})}}+{\frac {7-4{\sqrt {3}}}{\operatorname {M} (1,7-4{\sqrt {3}})}}\right)}$

where ${\displaystyle \operatorname {E} (k)}$ is the complete elliptic integral of the second kind with modulus ${\displaystyle k}$, ${\displaystyle \operatorname {M} }$ is the arithmetic–geometric mean and ${\displaystyle '}$ denotes the derivative with respect to the second variable.^[2]