Top Qs
Timeline
Chat
Perspective
Rectified 8-simplexes
From Wikipedia, the free encyclopedia
Remove ads
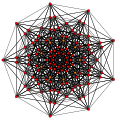
In eight-dimensional geometry, a rectified 8-simplex is a convex uniform 8-polytope, being a rectification of the regular 8-simplex.
There are unique 3 degrees of rectifications in regular 8-polytopes. Vertices of the rectified 8-simplex are located at the edge-centers of the 8-simplex. Vertices of the birectified 8-simplex are located in the triangular face centers of the 8-simplex. Vertices of the trirectified 8-simplex are located in the tetrahedral cell centers of the 8-simplex.
Remove ads
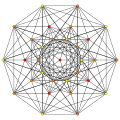
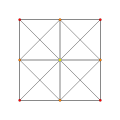
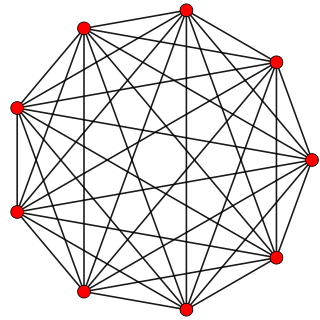
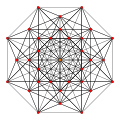
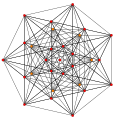
Rectified 8-simplex
Summarize
Perspective
| Rectified 8-simplex | |
|---|---|
| Type | uniform 8-polytope |
| Coxeter symbol | 061 |
| Schläfli symbol | t1{37} r{37} = {36,1} or |
| Coxeter-Dynkin diagrams | or |
| 7-faces | 18 |
| 6-faces | 108 |
| 5-faces | 336 |
| 4-faces | 630 |
| Cells | 756 |
| Faces | 588 |
| Edges | 252 |
| Vertices | 36 |
| Vertex figure | 7-simplex prism, {}×{3,3,3,3,3} |
| Petrie polygon | enneagon |
| Coxeter group | A8, [37], order 362880 |
| Properties | convex |
E. L. Elte identified it in 1912 as a semiregular polytope, labeling it as S1
8. It is also called 06,1 for its branching Coxeter-Dynkin diagram, shown as ![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]() . Acronym: rene (Jonathan Bowers)[1]
. Acronym: rene (Jonathan Bowers)[1]
The rectified 8-simplex is the vertex figure of the 9-demicube, and the edge figure of the uniform 261 honeycomb.
Coordinates
The Cartesian coordinates of the vertices of the rectified 8-simplex can be most simply positioned in 9-space as permutations of (0,0,0,0,0,0,0,1,1). This construction is based on facets of the rectified 9-orthoplex.
Images
Remove ads
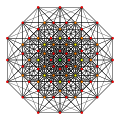
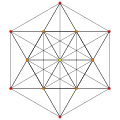
Birectified 8-simplex
Summarize
Perspective
E. L. Elte identified it in 1912 as a semiregular polytope, labeling it as S2
8. It is also called 05,2 for its branching Coxeter-Dynkin diagram, shown as ![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]() . Acronym: brene (Jonathan Bowers)[2]
. Acronym: brene (Jonathan Bowers)[2]
The birectified 8-simplex is the vertex figure of the 152 honeycomb.
Coordinates
The Cartesian coordinates of the vertices of the birectified 8-simplex can be most simply positioned in 9-space as permutations of (0,0,0,0,0,0,1,1,1). This construction is based on facets of the birectified 9-orthoplex.
Images
Remove ads
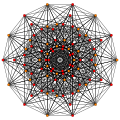
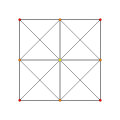
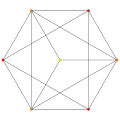
Trirectified 8-simplex
Summarize
Perspective
E. L. Elte identified it in 1912 as a semiregular polytope, labeling it as S3
8. It is also called 04,3 for its branching Coxeter-Dynkin diagram, shown as ![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]() . Acronym: trene (Jonathan Bowers)[3]
. Acronym: trene (Jonathan Bowers)[3]
Coordinates
The Cartesian coordinates of the vertices of the trirectified 8-simplex can be most simply positioned in 9-space as permutations of (0,0,0,0,0,1,1,1,1). This construction is based on facets of the trirectified 9-orthoplex.
Images
Related polytopes
The three presented polytopes are in the family of 135 uniform 8-polytopes with A8 symmetry.
Remove ads
Notes
References
External links
Wikiwand - on
Seamless Wikipedia browsing. On steroids.
Remove ads







 ...
...




 ...
...