Transpose

Matrix operation which flips a matrix over its diagonal / From Wikipedia, the free encyclopedia

Dear Wikiwand AI, let's keep it short by simply answering these key questions:

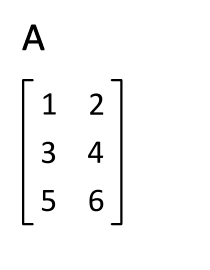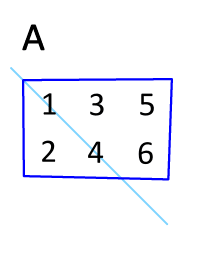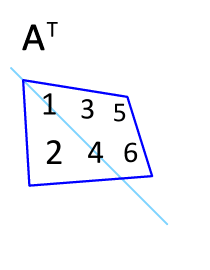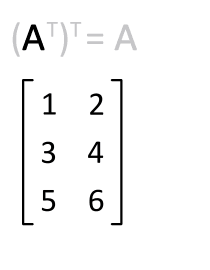
Can you list the top facts and stats about Transpose?

Summarize this article for a 10 years old

SHOW ALL QUESTIONS

In linear algebra, the transpose of a matrix is an operator which flips a matrix over its diagonal; that is, it switches the row and column indices of the matrix $A$ by producing another matrix, often denoted by $A T$ (among other notations).[1]

The transpose A^T of a matrix A can be obtained by reflecting the elements along its main diagonal. Repeating the process on the transposed matrix returns the elements to their original position.

The transpose of a matrix was introduced in 1858 by the British mathematician Arthur Cayley.[2] In the case of a logical matrix representing a binary relation R, the transpose corresponds to the converse relation R^T.

Oops something went wrong: