Truncated 5-simplexes

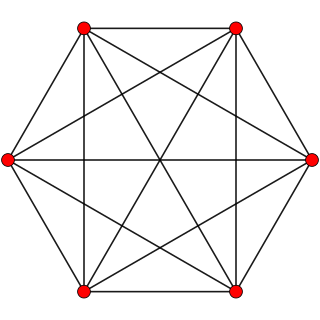
In five-dimensional geometry, a truncated 5-simplex is a convex uniform 5-polytope, being a truncation of the regular 5-simplex.

5-simplex	Truncated 5-simplex	Bitruncated 5-simplex
Orthogonal projections in A5 Coxeter plane

There are unique 2 degrees of truncation. Vertices of the truncation 5-simplex are located as pairs on the edge of the 5-simplex. Vertices of the bitruncation 5-simplex are located on the triangular faces of the 5-simplex.

Truncated 5-simplex

Truncated 5-simplex
Type	Uniform 5-polytope
Schläfli symbol	t{3,3,3,3}
Coxeter-Dynkin diagram
4-faces	12	6 {3,3,3} 6 t{3,3,3}
Cells	45	30 {3,3} 15 t{3,3}
Faces	80	60 {3} 20 {6}
Edges	75
Vertices	30
Vertex figure	( )v{3,3}
Coxeter group	A5 [3,3,3,3], order 720
Properties	convex

The truncated 5-simplex has 30 vertices, 75 edges, 80 triangular faces, 45 cells (15 tetrahedral, and 30 truncated tetrahedron), and 12 4-faces (6 5-cell and 6 truncated 5-cells).

Alternate names

• Truncated hexateron (Acronym: tix) (Jonathan Bowers)^[1]

Coordinates

The vertices of the truncated 5-simplex can be most simply constructed on a hyperplane in 6-space as permutations of (0,0,0,0,1,2) or of (0,1,2,2,2,2). These coordinates come from facets of the truncated 6-orthoplex and bitruncated 6-cube respectively.

Images

orthographic projections

Ak Coxeter plane	A5	A4
Graph
Dihedral symmetry	[6]	[5]
Ak Coxeter plane	A3	A2
Graph
Dihedral symmetry	[4]	[3]

Bitruncated 5-simplex

bitruncated 5-simplex
Type	Uniform 5-polytope
Schläfli symbol	2t{3,3,3,3}
Coxeter-Dynkin diagram
4-faces	12	6 2t{3,3,3} 6 t{3,3,3}
Cells	60	45 {3,3} 15 t{3,3}
Faces	140	80 {3} 60 {6}
Edges	150
Vertices	60
Vertex figure	{ }v{3}
Coxeter group	A5 [3,3,3,3], order 720
Properties	convex

Alternate names

• Bitruncated hexateron (Acronym: bittix) (Jonathan Bowers)^[2]

Coordinates

The vertices of the bitruncated 5-simplex can be most simply constructed on a hyperplane in 6-space as permutations of (0,0,0,1,2,2) or of (0,0,1,2,2,2). These represent positive orthant facets of the bitruncated 6-orthoplex, and the tritruncated 6-cube respectively.

Images

orthographic projections

Ak Coxeter plane	A5	A4
Graph
Dihedral symmetry	[6]	[5]
Ak Coxeter plane	A3	A2
Graph
Dihedral symmetry	[4]	[3]

Related uniform 5-polytopes

The truncated 5-simplex is one of 19 uniform 5-polytopes based on the [3,3,3,3] Coxeter group, all shown here in A₅ Coxeter plane orthographic projections. (Vertices are colored by projection overlap order, red, orange, yellow, green, cyan, blue, purple having progressively more vertices)

A5 polytopes
t0	t1	t2	t0,1	t0,2	t1,2	t0,3
t1,3	t0,4	t0,1,2	t0,1,3	t0,2,3	t1,2,3	t0,1,4
t0,2,4	t0,1,2,3	t0,1,2,4	t0,1,3,4	t0,1,2,3,4