Top Qs
Timeline
Chat
Perspective
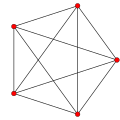
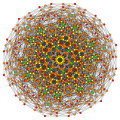
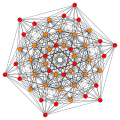
1 22 polytope
Uniform 6-polytope From Wikipedia, the free encyclopedia
Remove ads
In 6-dimensional geometry, the 122 polytope is a uniform polytope, constructed from the E6 group. It was first published in E. L. Elte's 1912 listing of semiregular polytopes, named as V72 (for its 72 vertices).[1]
Its Coxeter symbol is 122, describing its bifurcating Coxeter-Dynkin diagram, with a single ring on the end of the 1-node sequence. There are two rectifications of the 122, constructed by positions points on the elements of 122. The rectified 122 is constructed by points at the mid-edges of the 122. The birectified 122 is constructed by points at the triangle face centers of the 122.
These polytopes are from a family of 39 convex uniform polytopes in 6-dimensions, made of uniform polytope facets and vertex figures, defined by all permutations of rings in this Coxeter-Dynkin diagram: ![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]() .
.
Remove ads
122 polytope
Summarize
Perspective
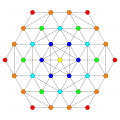
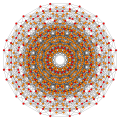
The 122 polytope contains 72 vertices, and 54 5-demicubic facets. It has a birectified 5-simplex vertex figure. Its 72 vertices represent the root vectors of the simple Lie group E6.
Alternate names
- Pentacontatetrapeton (Acronym: mo) - 54-facetted polypeton (Jonathan Bowers)[2]
Images
Construction
It is created by a Wythoff construction upon a set of 6 hyperplane mirrors in 6-dimensional space.
The facet information can be extracted from its Coxeter-Dynkin diagram, ![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]() .
.
Removing the node on either of 2-length branches leaves the 5-demicube, 121, ![]()
![]()
![]()
![]()
![]()
![]()
![]() .
.
The vertex figure is determined by removing the ringed node and ringing the neighboring node. This makes the birectified 5-simplex, 022, ![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]() .
.
Seen in a configuration matrix, the element counts can be derived by mirror removal and ratios of Coxeter group orders.[3]
Related complex polyhedron

The regular complex polyhedron 3{3}3{4}2, ![]()
![]()
![]()
![]()
![]() , in has a real representation as the 122 polytope in 4-dimensional space. It has 72 vertices, 216 3-edges, and 54 3{3}3 faces. Its complex reflection group is 3[3]3[4]2, order 1296. It has a half-symmetry quasiregular construction as
, in has a real representation as the 122 polytope in 4-dimensional space. It has 72 vertices, 216 3-edges, and 54 3{3}3 faces. Its complex reflection group is 3[3]3[4]2, order 1296. It has a half-symmetry quasiregular construction as ![]()
![]()
![]()
![]()
![]() , as a rectification of the Hessian polyhedron,
, as a rectification of the Hessian polyhedron, ![]()
![]()
![]()
![]()
![]() .[4]
.[4]
Related polytopes and honeycomb
Along with the semiregular polytope, 221, it is also one of a family of 39 convex uniform polytopes in 6-dimensions, made of uniform polytope facets and vertex figures, defined by all permutations of rings in this Coxeter-Dynkin diagram: ![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]() .
.
Geometric folding
The 122 is related to the 24-cell by a geometric folding E6 → F4 of Coxeter-Dynkin diagrams, E6 corresponding to 122 in 6 dimensions, F4 to the 24-cell in 4 dimensions. This can be seen in the Coxeter plane projections. The 24 vertices of the 24-cell are projected in the same two rings as seen in the 122.
Tessellations
This polytope is the vertex figure for a uniform tessellation of 6-dimensional space, 222, ![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]() .
.
Remove ads
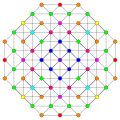
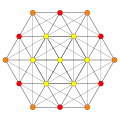
Rectified 122 polytope
Summarize
Perspective
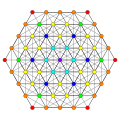
The rectified 122 polytope (also called 0221) can tessellate 6-dimensional space as the Voronoi cell of the E6* honeycomb lattice (dual of E6 lattice).[5]
Alternate names
- Birectified 221 polytope
- Rectified pentacontatetrapeton (Acronym: ram) - rectified 54-facetted polypeton (Jonathan Bowers)[6]
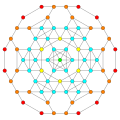
Images
Vertices are colored by their multiplicity in this projection, in progressive order: red, orange, yellow.
Construction
Its construction is based on the E6 group and information can be extracted from the ringed Coxeter-Dynkin diagram representing this polytope: ![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]() .
.
Removing the ring on the short branch leaves the birectified 5-simplex, ![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]() .
.
Removing the ring on either of 2-length branches leaves the birectified 5-orthoplex in its alternated form: t2(211), ![]()
![]()
![]()
![]()
![]()
![]()
![]() .
.
The vertex figure is determined by removing the ringed node and ringing the neighboring ring. This makes 3-3 duoprism prism, {3}×{3}×{}, ![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]() .
.
Seen in a configuration matrix, the element counts can be derived by mirror removal and ratios of Coxeter group orders.[3][6]
Remove ads
Truncated 122 polytope
Alternate names
- Truncated 122 polytope (Acronym: tim)[7]
Construction
Its construction is based on the E6 group and information can be extracted from the ringed Coxeter-Dynkin diagram representing this polytope: ![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]() .
.
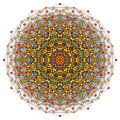
Images
Vertices are colored by their multiplicity in this projection, in progressive order: red, orange, yellow.
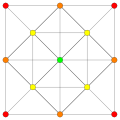
Birectified 122 polytope
Alternate names
- Bicantellated 221
- Birectified pentacontatetrapeton (barm) (Jonathan Bowers)[8]
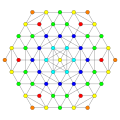
Images
Vertices are colored by their multiplicity in this projection, in progressive order: red, orange, yellow.
Remove ads
Trirectified 122 polytope
Alternate names
- Tricantellated 221
- Trirectified pentacontatetrapeton (Acronym: trim, old: cacam, tram, mak) (Jonathan Bowers)[9]
Remove ads
See also
Notes
References
Wikiwand - on
Seamless Wikipedia browsing. On steroids.
Remove ads











 ...
...