Arko (geometrio)

En geometrio, arko estas fermita segmento de diferencialebla linio en la du-dimensia ebeno; ekzemple, cirkla arko estas segmento de la perimetro (cirkonferenco) de cirklo. Se la arka segmento estas segmento de sfera ĉefcirklo (aŭ de granda elipso sur elipsoido), ĝi estas konsiderata grand-arka segmento.

Ĉi tiu artikolo temas pri dudimensia geometria objekto. Koncerne aliajn signifojn aliru la apartigilon Arko.

Cirkla sektoro A montrita en verda estas de radiuso r, angulo θ kaj longo L laŭ la cirkla perimetro

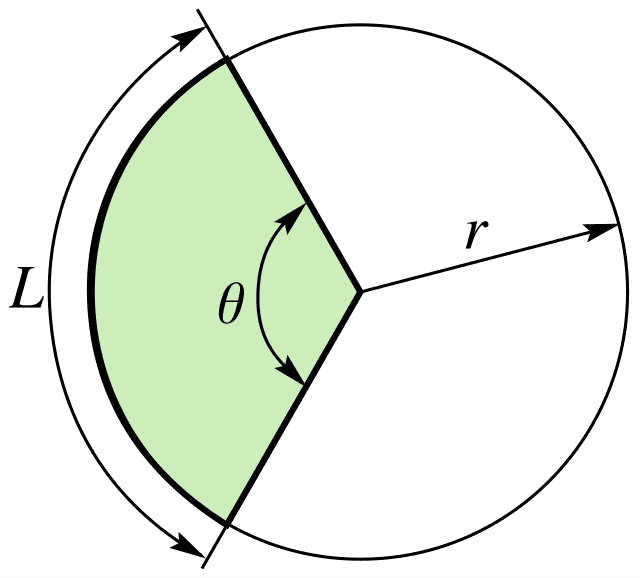
La longo de arko de cirklo kun radiuso r kaj substreĉanta angulo θ (mezurita en radianoj el centro de la cirklo, kio estas, la centra angulo) egalas al θr. Ĉi tio estas ĉar

${\displaystyle {\frac {L}{C}}={\frac {\theta }{2\pi }}\!}$

kie C estas la cirkonferenco. Anstataŭigante la cirkonferencon

${\displaystyle {\frac {L}{2\pi r}}={\frac {\theta }{2\pi }}\!}$

kaj solvanta por arka longo L rezultiĝas

L = θr

Por angulo α mezurita en gradoj, la amplekso en radianoj estas donita kiel

${\displaystyle \theta ={\frac {\alpha }{180}}\pi \!}$

kaj do la arka longo estas

${\displaystyle L={\frac {\alpha \pi r}{180}}\!}$

Vidu ankaŭ

• Arka longo
• Arko - aliaj signifoj de la termino arko
• Cirklo-arka grafeo
• Rekta segmento estas arko de rekto
• Sago (geometrio)

Eksteraj ligiloj

• Kategorio Arko (geometrio) en la Vikimedia Komunejo (Multrimedaj datumoj)

• Difino kaj propraĵoj de cirkla arko Kun interaga animacio
• A kolekto de paĝoj difinanta (arkoj, eĝoj) kaj iliaj propraĵoj, kun animis apletoj (Arkoj, Eĝoj), arka centra angulo, arka flankaparata angulo, centra angula teoremo kaj aliaj.
• Eric W. Weisstein, Arko en MathWorld.