Cube

For other uses, see Cube (disambiguation).

A cube or regular hexahedron is a three-dimensional solid object in geometry, which is bounded by six congruent square faces, a type of polyhedron. It has twelve congruent edges and eight vertices. It is a type of parallelepiped, with pairs of parallel opposite faces, and more specifically a rhombohedron, with congruent edges, and a rectangular cuboid, with right angles between pairs of intersecting faces and pairs of intersecting edges. It is an example of many classes of polyhedra: Platonic solid, regular polyhedron, parallelohedron, zonohedron, and plesiohedron. The dual polyhedron of a cube is the regular octahedron.

Cube
Type	Hanner polytope, orthogonal polyhedron, parallelohedron, Platonic solid, plesiohedron, regular polyhedron, zonohedron
Faces	6
Edges	12
Vertices	8
Vertex configuration	${\displaystyle 8\times (4^{3})}$
Schläfli symbol	${\displaystyle \{4,3\}}$
Symmetry group	octahedral symmetry ${\displaystyle \mathrm {O} _{\mathrm {h} }}$
Volume	side3
Dihedral angle (degrees)	90°
Dual polyhedron	regular octahedron
Properties	convex, edge-transitive, face-transitive, non-composite, orthogonal faces, vertex-transitive

The cube can be represented in many ways, one of which is the graph known as the cubical graph. It can be constructed by using the Cartesian product of graphs. The cube is the three-dimensional hypercube, a family of polytopes also including the two-dimensional square and four-dimensional tesseract. A cube with unit side length is the canonical unit of volume in three-dimensional space, relative to which other solid objects are measured. Other related figures involve the construction of polyhedra, space-filling and honeycombs, polycubes, as well as cubes in compounds, spherical, and topological space.

The cube was discovered in antiquity, associated with the nature of earth by Plato, for whom the Platonic solids are named. It can be derived differently to create more polyhedra, and it has applications to construct a new polyhedron by attaching others. Other applications include popular culture of toys and games, arts, optical illusions, architectural buildings, as well as natural science and technology.

Properties

3D model of a cube

A cube is a special case of rectangular cuboid in which the edges are equal in length.^[1] Like other cuboids, every face of a cube has four vertices, each of which connects with three congruent lines. These edges form square faces, making the dihedral angle of a cube between every two adjacent squares the interior angle of a square, 90°. Hence, the cube has six faces, twelve edges, and eight vertices. ^[2] Because of such properties, it is categorized as one of the five Platonic solids, a polyhedron in which all the regular polygons are congruent and the same number of faces meet at each vertex.^[3] Every three square faces surrounding a vertex is orthogonal each other, so the cube is classified as orthogonal polyhedron.^[4] The cube may also be considered as the parallelepiped in which all of its edges are equal^[5] (or more specifically a rhombohedron with congruent edges),^[6] and as the trigonal trapezohedron since its square faces are the rhombi' special case.^[7]

Measurement and other metric properties

A face diagonal in red and space diagonal in blue

Given a cube with edge length ${\displaystyle a}$. The face diagonal of a cube is the diagonal of a square ${\displaystyle a{\sqrt {2}}}$, and the space diagonal of a cube is a line connecting two vertices that is not in the same face, formulated as ${\displaystyle a{\sqrt {3}}}$. Both formulas can be determined by using Pythagorean theorem. The surface area of a cube ${\displaystyle A}$ is six times the area of a square:^[8] $${\displaystyle A=6a^{2}.}$$ The volume of a cuboid is the product of its length, width, and height. Because all the edges of a cube are equal in length, the formula for the volume of a cube as the third power of its side length, leading to the use of the term cubic to mean raising any number to the third power:^[9][8] $${\displaystyle V=a^{3}.}$$

Prince Rupert's cube

One special case is the unit cube, so named for measuring a single unit of length along each edge. It follows that each face is a unit square and that the entire figure has a volume of 1 cubic unit.^[10][11] Prince Rupert's cube, named after Prince Rupert of the Rhine, is the largest cube that can pass through a hole cut into the unit cube, despite having sides approximately 6% longer.^[12] A polyhedron that can pass through a copy of itself of the same size or smaller is said to have the Rupert property.^[13] A geometric problem of doubling the cube—alternatively known as the Delian problem—requires the construction of a cube with a volume twice the original by using a compass and straightedge solely. Ancient mathematicians could not solve this old problem until the French mathematician Pierre Wantzel in 1837 proved it was impossible.^[14]

The cube has three types of closed geodesics. The closed geodesics are paths on a cube's surface that are locally straight. In other words, they avoid the vertices of the polyhedron, follow line segments across the faces that they cross, and form complementary angles on the two incident faces of each edge that they cross. Two of its types are planar. The first type lies in a plane parallel to any face of the cube, forming a square, with the length being equal to the perimeter of a face, four times the length of each edge. The second type lies in a plane perpendicular to the long diagonal, forming a regular hexagon; its length is ${\displaystyle 3{\sqrt {2}}}$ times that of an edge. The third type is a non-planar hexagon.^[15]

Relation to the spheres

With edge length ${\displaystyle a}$, the inscribed sphere of a cube is the sphere tangent to the faces of a cube at their centroids, with radius ${\textstyle {\frac {1}{2}}a}$. The midsphere of a cube is the sphere tangent to the edges of a cube, with radius ${\textstyle {\frac {\sqrt {2}}{2}}a}$. The circumscribed sphere of a cube is the sphere tangent to the vertices of a cube, with radius ${\textstyle {\frac {\sqrt {3}}{2}}a}$.^[16]

For a cube whose circumscribed sphere has radius ${\displaystyle R}$, and for a given point in its three-dimensional space with distances ${\displaystyle d_{i}}$ from the cube's eight vertices, it is:^[17] $${\displaystyle {\frac {1}{8}}\sum _{i=1}^{8}d_{i}^{4}+{\frac {16R^{4}}{9}}=\left({\frac {1}{8}}\sum _{i=1}^{8}d_{i}^{2}+{\frac {2R^{2}}{3}}\right)^{2}.}$$

Symmetry

The cube has octahedral symmetry ${\displaystyle \mathrm {O} _{\mathrm {h} }}$. It is composed of reflection symmetry, a symmetry by cutting into two halves by a plane. There are nine reflection symmetries: the five are cut the cube from the midpoints of its edges, and the four are cut diagonally. It is also composed of rotational symmetry, a symmetry by rotating it around the axis, from which the appearance is interchangeable. It has octahedral rotation symmetry ${\displaystyle \mathrm {O} }$: three axes pass through the cube's opposite faces centroid, six through the cube's opposite edges midpoints, and four through the cube's opposite vertices; each of these axes is respectively four-fold rotational symmetry (0°, 90°, 180°, and 270°), two-fold rotational symmetry (0° and 180°), and three-fold rotational symmetry (0°, 120°, and 240°).^[18][19][20] Its automorphism group is the order of 48.^[21]

The dual polyhedron of a cube is the regular octahedron

The dual polyhedron can be obtained from each of the polyhedra's vertices tangent to a plane by the process known as polar reciprocation.^[22] One property of dual polyhedra is that the polyhedron and its dual share their three-dimensional symmetry point group. In this case, the dual polyhedron of a cube is the regular octahedron, and both of these polyhedron has the same symmetry, the octahedral symmetry.^[23]

The cube is face-transitive, meaning its two squares are alike and can be mapped by rotation and reflection.^[24] It is vertex-transitive, meaning all of its vertices are equivalent and can be mapped isometrically under its symmetry.^[25] It is also edge-transitive, meaning the same kind of faces surround each of its vertices in the same or reverse order, all two adjacent faces have the same dihedral angle. Therefore, the cube is regular polyhedron because it requires those properties.^[26] Each vertex is surrounded by three squares, so the cube is ${\displaystyle 4.4.4}$ by vertex configuration or ${\displaystyle \{4,3\}}$ in Schläfli symbol.^[27]

Applications

A six-sided dice

A completed Skewb

A sculpture Alamo

Cubes have appeared in many roles in popular culture. It is the most common form of dice.^[24] Puzzle toys such as pieces of a Soma cube,^[28] Rubik's Cube, and Skewb are built of cubes.^[29] Minecraft is an example of a sandbox video game of cubic blocks.^[30] The outdoor sculpture Alamo (1967) is a cube standing on a vertex.^[31] Optical illusions such as the impossible cube and Necker cube have been explored by artists such as M. C. Escher.^[32] Salvador Dalí's painting Corpus Hypercubus (1954) contains an unfolding of a tesseract into a six-armed cross; a similar construction is central to Robert A. Heinlein's short story "And He Built a Crooked House" (1940).^[33][34] The cube was applied in Alberti's treatise on Renaissance architecture, De re aedificatoria (1450).^[35] Kubuswoningen is known for a set of cubical houses in which its hexagonal space diagonal becomes the main floor.^[36]

Simple cubic crystal structure

Pyrite cubic crystals

Ball-and-stick model of cubane

Cubes are also found in natural science and technology. It is applied to the unit cell of a crystal known as a cubic crystal system.^[37] Pyrite is an example of a mineral with a commonly cubic shape, although there are many varied shapes.^[38] The radiolarian Lithocubus geometricus, discovered by Ernst Haeckel, has a cubic shape.^[39] A historical attempt to unify three physics ideas of relativity, gravitation, and quantum mechanics used the framework of a cube known as a cGh cube.^[40] Cubane is a synthetic hydrocarbon consisting of eight carbon atoms arranged at the corners of a cube, with one hydrogen atom attached to each carbon atom.^[41] Other technological cubes include the spacecraft device CubeSat,^[42] and thermal radiation demonstration device Leslie cube.^[43] Cubical grids are usual in three-dimensional Cartesian coordinate systems.^[44] In computer graphics, an algorithm divides the input volume into a discrete set of cubes known as the unit on isosurface,^[45] and the faces of a cube can be used for mapping a shape.^[46]

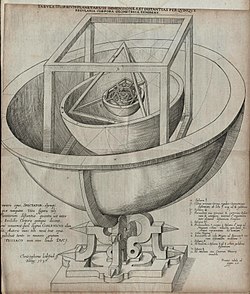
Sketch of a cube by Johannes Kepler

Kepler's Platonic solid model of the Solar System

The Platonic solids are five polyhedra known since antiquity. The set is named for Plato who, in his dialogue Timaeus, attributed these solids to nature. One of them, the cube, represented the classical element of earth because of its stability.^[47] Euclid's Elements defined the Platonic solids, including the cube, and showed how to find the ratio of the circumscribed sphere's diameter to the edge length.^[48] Following Plato's use of the regular polyhedra as symbols of nature, Johannes Kepler in his Harmonices Mundi sketched each of the Platonic solids; he decorated ane side of the cube with a tree.^[47] In his Mysterium Cosmographicum, Kepler also proposed that the ratios between sizes of the orbits of the planets are the ratios between the sizes of the inscribed and circumscribed spheres of the Platonic solids. That is, if the orbits are great circles on spheres, the sphere of Mercury is tangent to a regular octahedron, whose vertices lie on the sphere of Venus, which is in turn tangent to a regular icosahedron, within the sphere of Earth, within a regular dodecahedron, within the sphere of Mars, within a regular tetrahedron, within the sphere of Jupiter, within a cube, within the sphere of Saturn. In fact the orbits are not circles but ellipses (as Kepler himself later showed), and these relations are only approximate.^[49]

Construction

Nets of a cube

An elementary way to construct is using its net, an arrangement of edge-joining polygons, constructing a polyhedron by connecting along the edges of those polygons. Eleven nets for the cube are shown here.^[50]

In analytic geometry, a cube may be constructed using the Cartesian coordinate systems. For a cube centered at the origin, with edges parallel to the axes and with an edge length of 2, the Cartesian coordinates of the vertices are ${\displaystyle (\pm 1,\pm 1,\pm 1)}$.^[51] Its interior consists of all points ${\displaystyle (x_{0},x_{1},x_{2})}$ with ${\displaystyle -1<x_{i}<1}$ for all ${\displaystyle i}$. A cube's surface with center ${\displaystyle (x_{0},y_{0},z_{0})}$ and edge length of ${\displaystyle 2a}$ is the locus of all points ${\displaystyle (x,y,z)}$ such that $${\displaystyle \max\{|x-x_{0}|,|y-y_{0}|,|z-z_{0}|\}=a.}$$

The cube is Hanner polytope, because it can be constructed by using Cartesian product of three line segments. Its dual polyhedron, the regular octahedron, is constructed by direct sum of three line segments.^[52]

Representation

As a graph

Main article: Hypercube graph

The graph of a cube

According to Steinitz's theorem, the graph can be represented as the skeleton of a polyhedron; roughly speaking, a framework of a polyhedron. Such a graph has two properties: planar (the edges of a graph are connected to every vertex without crossing other edges), and 3-connected (whenever a graph with more than three vertices, and two of the vertices are removed, the edges remain connected).^[53][54] The skeleton of a cube can be represented as the graph, and it is called the cubical graph, a Platonic graph. It has the same number of vertices and edges as the cube, twelve vertices and eight edges.^[55] The cubical graph is also classified as a prism graph, resembling the skeleton of a cuboid.^[56]

The cubical graph is a special case of hypercube graph or ${\displaystyle n}$-cube—denoted as ${\displaystyle Q_{n}}$—because it can be constructed by using the operation known as the Cartesian product of graphs: it involves two graphs connecting the pair of vertices with an edge to form a new graph.^[57] In the case of the cubical graph, it is the product of two ${\displaystyle Q_{2}}$; roughly speaking, it is a graph resembling a square. In other words, the cubical graph is constructed by connecting each vertex of two squares with an edge. Notationally, the cubical graph is ${\displaystyle Q_{3}}$.^[58] Like any hypercube graph, it has a cycle visits every vertex exactly once,^[59] and it is also an example of a unit distance graph.^[60]

The cubical graph is bipartite, meaning every independent set of four vertices can be disjoint and the edges connected in those sets.^[61] However, every vertex in one set cannot connect all vertices in the second, so this bipartite graph is not complete.^[62] It is an example of both crown graph and bipartite Kneser graph.^[63][61]

In orthogonal projection

An object illuminated by parallel rays of light casts a shadow on a plane perpendicular to those rays, called an orthogonal projection. A polyhedron is considered equiprojective if, for some position of the light, its orthogonal projection is a regular polygon. The cube is equiprojective because, if the light is parallel to one of the four lines joining a vertex to the opposite vertex, its projection is a regular hexagon.^[64]

As a configuration matrix

The cube can be represented as configuration matrix. A configuration matrix is a matrix in which the rows and columns correspond to the elements of a polyhedron as in the vertices, edges, and faces. The diagonal of a matrix denotes the number of each element that appears in a polyhedron, whereas the non-diagonal of a matrix denotes the number of the column's elements that occur in or at the row's element. As mentioned above, the cube has eight vertices, twelve edges, and six faces; each element in a matrix's diagonal is denoted as 8, 12, and 6. The first column of the middle row indicates that there are two vertices in (i.e., at the extremes of) each edge, denoted as 2; the middle column of the first row indicates that three edges meet at each vertex, denoted as 3. The following matrix is:^[65] $${\displaystyle {\begin{bmatrix}{\begin{matrix}8&3&3\\2&12&2\\4&4&6\end{matrix}}\end{bmatrix}}}$$

Related figures

Construction of polyhedra

Some of the derived cubes, the stellated octahedron and tetrakis hexahedron.

The cube can appear in the construction of a polyhedron, and some of its types can be derived differently in the following:

• When faceting a cube, meaning removing part of the polygonal faces without creating new vertices of a cube, the resulting polyhedron is the stellated octahedron.^[66]
• The cube is non-composite polyhedron, meaning it is a convex polyhedron that cannot be separated into two or more regular polyhedra. The cube can be applied to construct a new convex polyhedron by attaching another.^[67] Attaching a square pyramid to each square face of a cube produces its Kleetope, a polyhedron known as the tetrakis hexahedron.^[68] Suppose one and two equilateral square pyramids are attached to their square faces. In that case, they are the construction of an elongated square pyramid and elongated square bipyramid respectively, the Johnson solid's examples.^[69]
• Each of the cube's vertices can be truncated, and the resulting polyhedron is the Archimedean solid, the truncated cube.^[70] When its edges are truncated, it is a rhombicuboctahedron.^[71] Relatedly, the rhombicuboctahedron can also be constructed by separating the cube's faces and then spreading away, after which adding other triangular and square faces between them; this is known as the "expanded cube". Similarly, it is constructed by the cube's dual, the regular octahedron.^[72]
• The barycentric subdivision of a cube (or its dual, the regular octahedron) is the disdyakis dodecahedron, a Catalan solid.^[73]
• The corner region of a cube can also be truncated by a plane (e.g., spanned by the three neighboring vertices), resulting in a trirectangular tetrahedron.^[74]
• The snub cube is an Archimedean solid that can be constructed by separating away the cube square's face, and filling their gaps with twisted angle equilateral triangles, a process known as snub.^[75]

The cube can be constructed with six square pyramids, tiling space by attaching their apices. In some cases, this produces the rhombic dodecahedron circumscribing a cube.^[76][77]

Polycubes

Main article: Polycubes

Dali cross, the net of a tesseract

Polycube is a polyhedron in which the faces of many cubes are attached. Analogously, it can be interpreted as the polyominoes in three-dimensional space.^[78] When four cubes are stacked vertically, and the other four are attached to the second-from-top cube of the stack, the resulting polycube is Dali cross, after Salvador Dali. In addition to popular cultures, the Dali cross is a tile space polyhedron,^[79][80] which can be represented as the net of a tesseract. A tesseract is a cube analogous' four-dimensional space bounded by twenty-four squares and eight cubes.^[81]

Space-filling and honeycombs

Hilbert's third problem asked whether every two equal-volume polyhedra could always be dissected into polyhedral pieces and reassembled into each other. If it were, then the volume of any polyhedron could be defined axiomatically as the volume of an equivalent cube into which it could be reassembled. Max Dehn solved this problem in an invention Dehn invariant, answering that not all polyhedra can be reassembled into a cube.^[82] It showed that two equal volume polyhedra should have the same Dehn invariant, except for the two tetrahedra whose Dehn invariants were different.^[83]

Cubic honeycomb

The cube has a Dehn invariant of zero. This indicates the cube is applied for honeycomb. More strongly, the cube is a space-filling tile in three-dimensional space in which the construction begins by attaching a polyhedron onto its faces without leaving a gap.^[84] The cube is a plesiohedron, a special kind of space-filling polyhedron that can be defined as the Voronoi cell of a symmetric Delone set.^[85] The plesiohedra include the parallelohedra, which can be translated without rotating to fill a space in which each face of any of its copies is attached to a like face of another copy. There are five kinds of parallelohedra, one of which is the cuboid.^[86] Every three-dimensional parallelohedron is zonohedron, a centrally symmetric polyhedron whose faces are centrally symmetric polygons.^[87] In the case of cube, it can be represented as the cell. Some honeycombs have cubes as the only cells; one example is cubic honeycomb, the only regular honeycomb in Euclidean three-dimensional space, having four cubes around each edge.^[88][89]

Miscellaneous

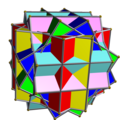
Enumeration according to Skilling (1976): compound of six cubes with rotational freedom ${\displaystyle \mathrm {UC} _{7}}$, three cubes ${\displaystyle \mathrm {UC} _{8}}$, and five cubes ${\displaystyle \mathrm {UC} _{9}}$

Compound of cubes is the polyhedral compounds in which the cubes share the same centre. They belong to the uniform polyhedron compound, meaning they are polyhedral compounds whose constituents are identical (although possibly enantiomorphous) uniform polyhedra, in an arrangement that is also uniform. Respectively, the list of compounds enumerated by Skilling (1976) in the seventh to ninth uniform compounds for the compound of six cubes with rotational freedom, three cubes, and five cubes.^[90] Two compounds, consisting of two and three cubes were found in Escher's wood engraving print Stars and Max Brückner's book Vielecke und Vielflache.^[91]

Spherical cube

A view in three-dimensional torus

The spherical cube represents the spherical polyhedron, which can be modeled by the arc of great circles, creating bounds as the edges of a spherical square.^[92] Hence, the spherical cube consists of six spherical squares with 120° interior angles on each vertex. It has vector equilibrium, meaning that the distance from the centroid and each vertex is the same as the distance from that and each edge.^[93][94] Its dual is the spherical octahedron.^[92]

The topological object three-dimensional torus is a topological space defined to be homeomorphic to the Cartesian product of three circles. It can be represented as a three-dimensional model of the cube shape.^[95]

See also

• Bhargava cube, a configuration to study the law of binary quadratic form and other such forms, of which the cube's vertices represent the integer.
• Chazelle polyhedron, a notched opposite faces of a cube.
• Cubism, an art movement of revolutionized painting and the visual arts.
• Hemicube, an abstract polyhedron produced by identifying opposite faces of a cube
• Squaring the square's three-dimensional analogue, cubing the cube