Runcinated 5-orthoplexes

In five-dimensional geometry, a runcinated 5-orthoplex is a convex uniform 5-polytope with 3rd order truncation (runcination) of the regular 5-orthoplex.

5-orthoplex	Runcinated 5-orthoplex	Runcinated 5-cube
Runcitruncated 5-orthoplex	Runcicantellated 5-orthoplex	Runcicantitruncated 5-orthoplex
Runcitruncated 5-cube	Runcicantellated 5-cube	Runcicantitruncated 5-cube
Orthogonal projections in B5 Coxeter plane

There are 8 runcinations of the 5-orthoplex with permutations of truncations, and cantellations. Four are more simply constructed relative to the 5-cube.

Runcinated 5-orthoplex

Runcinated 5-orthoplex
Type	Uniform 5-polytope
Schläfli symbol	t0,3{3,3,3,4}
Coxeter-Dynkin diagram
4-faces	162
Cells	1200
Faces	2160
Edges	1440
Vertices	320
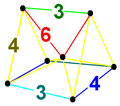
Vertex figure
Coxeter group	B5 [4,3,3,3] D5 [32,1,1]
Properties	convex

Alternate names

• Runcinated pentacross
• Small prismated triacontiditeron (Acronym: spat) (Jonathan Bowers)^[1]

Coordinates

The vertices of the can be made in 5-space, as permutations and sign combinations of:

(0,1,1,1,2)

Images

orthographic projections

Coxeter plane	B5	B4 / D5	B3 / D4 / A2
Graph
Dihedral symmetry	[10]	[8]	[6]
Coxeter plane	B2	A3
Graph
Dihedral symmetry	[4]	[4]

Runcitruncated 5-orthoplex

Runcitruncated 5-orthoplex
Type	uniform 5-polytope
Schläfli symbol	t0,1,3{3,3,3,4} t0,1,3{3,31,1}
Coxeter-Dynkin diagrams
4-faces	162
Cells	1440
Faces	3680
Edges	3360
Vertices	960
Vertex figure
Coxeter groups	B5, [3,3,3,4] D5, [32,1,1]
Properties	convex

Alternate names

• Runcitruncated pentacross
• Prismatotruncated triacontiditeron (Acronym: pattit) (Jonathan Bowers)^[2]

Coordinates

Cartesian coordinates for the vertices of a runcitruncated 5-orthoplex, centered at the origin, are all 80 vertices are sign (4) and coordinate (20) permutations of

(±3,±2,±1,±1,0)

Images

orthographic projections

Coxeter plane	B5	B4 / D5	B3 / D4 / A2
Graph
Dihedral symmetry	[10]	[8]	[6]
Coxeter plane	B2	A3
Graph
Dihedral symmetry	[4]	[4]

Runcicantellated 5-orthoplex

Runcicantellated 5-orthoplex
Type	Uniform 5-polytope
Schläfli symbol	t0,2,3{3,3,3,4} t0,2,3{3,3,31,1}
Coxeter-Dynkin diagram
4-faces	162
Cells	1200
Faces	2960
Edges	2880
Vertices	960
Vertex figure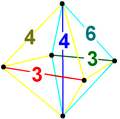
Coxeter group	B5 [4,3,3,3] D5 [32,1,1]
Properties	convex

Alternate names

• Runcicantellated pentacross
• Prismatorhombated triacontiditeron (Acronym: pirt) (Jonathan Bowers)^[3]

Coordinates

The vertices of the runcicantellated 5-orthoplex can be made in 5-space, as permutations and sign combinations of:

(0,1,2,2,3)

Images

orthographic projections

Coxeter plane	B5	B4 / D5	B3 / D4 / A2
Graph
Dihedral symmetry	[10]	[8]	[6]
Coxeter plane	B2	A3
Graph
Dihedral symmetry	[4]	[4]

Runcicantitruncated 5-orthoplex

Runcicantitruncated 5-orthoplex
Type	Uniform 5-polytope
Schläfli symbol	t0,1,2,3{3,3,3,4}
Coxeter-Dynkin diagram
4-faces	162
Cells	1440
Faces	4160
Edges	4800
Vertices	1920
Vertex figure	Irregular 5-cell
Coxeter groups	B5 [4,3,3,3] D5 [32,1,1]
Properties	convex, isogonal

Alternate names

• Runcicantitruncated pentacross
• Great prismated triacontiditeron (gippit) (Jonathan Bowers)^[4]

Coordinates

The Cartesian coordinates of the vertices of a runcicantitruncated 5-orthoplex having an edge length of √2 are given by all permutations of coordinates and sign of:

${\displaystyle \left(0,1,2,3,4\right)}$

Images

orthographic projections

Coxeter plane	B5	B4 / D5	B3 / D4 / A2
Graph
Dihedral symmetry	[10]	[8]	[6]
Coxeter plane	B2	A3
Graph
Dihedral symmetry	[4]	[4]

Snub 5-demicube

The snub 5-demicube defined as an alternation of the omnitruncated 5-demicube is not uniform, but it can be given Coxeter diagram or and symmetry [3^2,1,1]⁺ or [4,(3,3,3)⁺], and constructed from 10 snub 24-cells, 32 snub 5-cells, 40 snub tetrahedral antiprisms, 80 2-3 duoantiprisms, and 960 irregular 5-cells filling the gaps at the deleted vertices.

Related polytopes

This polytope is one of 31 uniform 5-polytopes generated from the regular 5-cube or 5-orthoplex.

B5 polytopes
β5	t1β5	t2γ5	t1γ5	γ5	t0,1β5	t0,2β5	t1,2β5
t0,3β5	t1,3γ5	t1,2γ5	t0,4γ5	t0,3γ5	t0,2γ5	t0,1γ5	t0,1,2β5
t0,1,3β5	t0,2,3β5	t1,2,3γ5	t0,1,4β5	t0,2,4γ5	t0,2,3γ5	t0,1,4γ5	t0,1,3γ5
t0,1,2γ5	t0,1,2,3β5	t0,1,2,4β5	t0,1,3,4γ5	t0,1,2,4γ5	t0,1,2,3γ5	t0,1,2,3,4γ5