Top Qs
Timeline
Chat
Perspective
Cardinality
Size of a set in mathematics From Wikipedia, the free encyclopedia
Remove ads
In mathematics, cardinality is an intrinsic property of sets, roughly meaning the number of individual objects they contain, which may be infinite. The concept is understood through one-to-one correspondences between sets. That is, if their objects can be paired such that each object has a pair, and no object is paired more than once.

The basic concepts of cardinality go back as early as the 6th century BCE, and there are several close encounters with it throughout history. However, it is considered to have been first introduced formally to mathematics by Georg Cantor at the turn of the 20th century. Cantor's theory of cardinality was then formalized, popularized, and explored by many influential mathematicians of the time, and has since become a fundamental concept of mathematics.
Two sets are said to be equinumerous or have the same cardinality if there exists a one-to-one correspondence between them. Otherwise, one is said to be strictly larger or strictly smaller than the other. A set is countably infinite if it can be placed in one-to-one correspondence with the set of natural numbers For example, the set of even numbers and the set of rational numbers are countable. Uncountable sets are those strictly larger than the set of natural numbers—for example, the set of all real numbers or the powerset of the set of natural numbers.
For finite sets, cardinality coincides with the natural number found by counting their elements. However, it is more often difficult to ascribe "sizes" to infinite sets. Thus, a system of cardinal numbers can be developed to extend the role of natural numbers as abstractions of the sizes of sets. The cardinal number corresponding to a set is written as between two vertical bars. Most commonly, the Aleph numbers are used, since it can be shown every infinite set has cardinality equivalent to some Aleph.
The set of natural numbers has cardinality . The question of whether the real numbers have cardinality is known as the continuum hypothesis, which has been shown to be unprovable in standard set theories such as Zermelo–Fraenkel set theory. Alternative set theories and additional axioms give rise to different properties and have often strange or unintuitive consequences. However, every theory of cardinality using standard logical foundations of mathematics admits Skolem's paradox, which roughly asserts that basic properties of cardinality are not absolute, but relative to the model in which the cardinality is measured.
Remove ads
Definition and etymology
Summarize
Perspective
Cardinality is an intrinsic property of sets which defines their size, roughly corresponding to the number of individual objects they contain.[1] Fundamentally however, it is different from the concepts of number or counting as the cardinalities of two sets can be compared without referring to their number of elements, or defining number at all. For example, in the image above, a set of apples is compared to a set of oranges such that every fruit is used exactly once which shows these two sets have the same cardinality, even if one doesn't know how many of each there are.[2][3] Thus, cardinality is measured by putting sets in one-to-one correspondence. If it is possible, the sets are said to have the same cardinality, and if not, one set is said to be strictly larger or strictly smaller than the other.[4]
Georg Cantor, the originator of the concept, defined cardinality as "the general concept which, with the aid of our intelligence, results from a set when we abstract from the nature of its various elements and from the order of their being given."[5] This definition was considered to be imprecise, unclear, and purely psychological.[6] Thus, cardinal numbers, a means of measuring cardinality, became the main way of presenting the concept. The distinction between the two is roughly analogous to the difference between an object's mass and its mass in kilograms.[7] However, somewhat confusingly, the phrases "The cardinality of M" and "The cardinal number of M" are used interchangeably.
The term cardinality originates from the post-classical Latin cardo ("to hinge"), which referred to something central or pivotal, both literally and metaphorically. This passed into medieval Latin and then into English, where cardinal came to describe things considered to be, in some sense, fundamental, such as, cardinal sins, cardinal directions, and (in lingustics) cardinal numbers.[8][9] The last of which referred to numbers used for counting (e.g., one, two, three),[10] as opposed to ordinal numbers, which express order (e.g., first, second, third),[11] and nominal numbers used for labeling without meaning (e.g., jersey numbers and serial numbers).[12]
In mathematics, the notion of cardinality was first introduced by Georg Cantor in the late 19th century, wherein he used the used the term Mächtigkeit, which may be translated as "magnitude" or "power", though Cantor credited the term to a work by Jakob Steiner on projective geometry.[13][14][15] The terms cardinality and cardinal number were eventually adopted from the grammatical sense, and later translations would use these terms.[16][17]
Remove ads
History
Summarize
Perspective
Ancient history

From the 6th century BCE, the writings of Greek philosophers, such as Anaximander, show hints of comparing infinite sets or shapes, however, it was generally viewed as paradoxical and imperfect (cf. Zeno's paradoxes).[18] Aristotle distinguished between the notions of actual infinity and potential infinity, arguing that Greek mathematicians understood the difference, and that they "do not need the [actual] infinite and do not use it."[19] The Greek notion of number (αριθμός, arithmos) was used exclusively for a definite number of definite objects (i.e. finite numbers).[20] This would be codified in Euclid's Elements, where the fifth common notion states "The whole is greater than the part", often called the Euclidean principle. This principle would be the dominating philosophy in mathematics until the 19th century.[18][21]
Around the 4th century BCE, Jaina mathematics would be the first to discuss different sizes of infinity. They defined three major classes of number: enumerable (finite numbers), unenumerable (asamkhyata, roughly, countably infinite), and infinite (ananta). Then they had five classes of infinite numbers: infinite in one direction, infinite in both directions, infinite in area, infinite everywhere, and infinite perpetually.[22][23]
One of the earliest explicit uses of a one-to-one correspondence is recorded in Aristotle's Mechanics (c. 350 BCE), known as Aristotle's wheel paradox. The paradox can be briefly described as follows: A wheel is depicted as two concentric circles. The larger, outer circle is tangent to a horizontal line (e.g. a road that it rolls on), while the smaller, inner circle is rigidly affixed to the larger. Assuming the larger circle rolls along the line without slipping (or skidding) for one full revolution, the distances moved by both circles are the same: the circumference of the larger circle. Further, the lines traced by the bottom-most point of each is the same length.[24] Since the smaller wheel does not skip any points, and no point on the smaller wheel is used more than once, there is a one-to-one correspondence between the two circles.[25][26][27]
Pre-Cantorian set theory
Galileo Galilei presented what was later coined Galileo's paradox in his book Two New Sciences (1638),[28] where he presents a seeming paradox in infinite sequences of numbers. It goes roughly as follows: for each perfect square 1, 4, 9, 16, and so on, there is a unique square root 1, 2, 3, 4, and so on. Therefore, there are as many perfect squares as there are square roots. However, every number is a square root, since it can be squared, but not every number is a perfect square. Moreover, the proportion of perfect squares as one passes larger values diminishes, and is eventually smaller than any given fraction. Galileo denied that this was fundamentally contradictory, however he concluded that this meant we could not compare the sizes of infinite sets, missing the opportunity to discover cardinality.[29]
In A Treatise of Human Nature (1739), David Hume is quoted for saying "When two numbers are so combined, as that the one has always a unit answering to every unit of the other, we pronounce them equal",[30] now called Hume's principle, which was used extensively by Gottlob Frege later during the rise of set theory.[31][32][33]
Bernard Bolzano's Paradoxes of the Infinite (Paradoxien des Unendlichen, 1851) is often considered the first systematic attempt to introduce the concept of sets into mathematical analysis. In this work, Bolzano defended the notion of actual infinity, presented an early formulation of what would later be recognized as one-to-one correspondence between infinite sets. He discussed examples such as the pairing between the intervals and by the relation and revisited Galileo's paradox. However, he too resisted saying that these sets were, in that sense, the same size. While Paradoxes of the Infinite anticipated several ideas central to later set theory, the work had little influence on contemporary mathematics, in part due to its posthumous publication and limited circulation.[34][35][36]
Early set theory
Georg Cantor

The concept of cardinality emerged nearly fully formed in the work of Georg Cantor during the 1870s and 1880s, in the context of mathematical analysis. In a series of papers beginning with On a Property of the Collection of All Real Algebraic Numbers (1874),[37] Cantor introduced the idea of comparing the sizes of infinite sets, through the notion of one-to-one correspondence.[38] He showed that the set of real numbers was, in this sense, strictly larger than the set of natural numbers using a nested intervals argument.[39] This result was later refined into the more widely known diagonal argument of 1891, published in Über eine elementare Frage der Mannigfaltigkeitslehre,[40] where he also proved the more general result (now called Cantor's Theorem) that the power set of any set is strictly larger than the set itself.[41]
Cantor introduced the notion cardinal numbers in terms of ordinal numbers. He viewed cardinal numbers as an abstraction of sets, introduced the notations, where, for a given set , the order type of that set was written , and the cardinal number was , a double abstraction.[42] He also introduced the Aleph sequence for infinite cardinal numbers. These notations appeared in correspondence and were formalized in his later writings, particularly the series Beiträge zur Begründung der transfiniten Mengenlehre (1895–1897).[43] In these works, Cantor developed an arithmetic of cardinal numbers, defining addition, multiplication, and exponentiation of cardinal numbers based on set-theoretic constructions. This led to the formulation of the Continuum Hypothesis (CH), the proposition that no set has cardinality strictly between the cardinality of the natural numbers and the cardinality of the continuum , that is whether . Cantor was unable to resolve CH and left it as an open problem.[44]
Other contributors
Parallel to Cantor’s development, Richard Dedekind independently formulated many advanced theorems of set theory, and helped establish set-theoretic foundations of algebra and arithmetic.[45] Dedekind's The Nature and Meaning of Numbers (1888)[46] emphasized structural properties over extensional definitions, and supported the bijective formulation of size and number. Dedekind was in correspondence with Cantor during the development of set theory; he supplied Cantor with a proof of the countability of the algebraic numbers, and gave feedback and modifications on Cantor's proofs before publishing.[47][34][48]
After Cantor's 1883 proof that all finite-dimensional spaces have the same cardinality,[49] in 1890, Giuseppe Peano introduced the Peano curve, which was a more visual proof that the unit interval has the same cardinality as the unit square on [50] This created a new area of mathematical analysis studying what is now called space-filling curves.[51]
German logician Gottlob Frege attempted to ground the concepts of number and arithmetic in logic using Cantor's theory of cardinality and Hume's principle in Die Grundlagen der Arithmetik (1884) and the subsequent Grundgesetze der Arithmetik (1893, 1903).[31][32][33] Frege defined cardinal numbers as equivalence classes of sets under equinumerosity. However, Frege's approach to set theory was later shown to be flawed. His approach was eventually reformalized by Bertrand Russell and Alfred Whitehead in Principia Mathematica (1910–1913, vol. II)[52] using a theory of types.[53] Though Russell initially had difficulties understanding Cantor's and Frege’s intuitions of cardinality.[54][55] This definition of cardinal numbers is now referred to as the Frege–Russell definition.[56] This definition was eventually superseded by the convention established by John von Neumann in 1928 which uses representatives to define cardinal numbers.[57]
At the Paris conference of the International Congress of Mathematicians in 1900, David Hilbert, one of the most influential mathematicians of the time, gave a speech wherein he presented ten unsolved problems (of a total of 23, later published, now called Hilbert's problems). Of these, he placed "Cantor's problem" (now called the Continuum Hypothesis) as the first on the list. This list of problems would prove to be very influential in 20th century mathematics, and attracted a lot of attention from other mathematicians toward Cantor's theory of cardinality.[58][34]
Axiomatic set theory
In 1908, Ernst Zermelo proposed the first axiomatization of set theory, now called Zermelo set theory, primarily to support his earlier (1904) proof of the Well-ordering theorem, which showed that all cardinal numbers could be represented as Alephs, though the proof required a controversial principle now known as the Axiom of Choice (AC).[59] Zermelo's system would later be extended by Abraham Fraenkel and Thoralf Skolem in the 1920s to create the standard foundation of set theory, called Zermelo–Fraenkel set theory (ZFC, "C" for the Axiom of Choice). ZFC provided a rigorous foundation through which infinite cardinals could be systematically studied while avoiding the paradoxes of naive set theory.[34][60]
Ignoring possible foundational issues, during the early 1900s, Felix Hausdorff would begin studying "exorbitant numbers": roughly, very large cardinal numbers, or what are now called inaccessible cardinals. This work would be continued and popularized by several other influential set theorists such as Paul Mahlo—who introduced Mahlo cardinals—as well as Wacław Sierpiński, and Alfred Tarski. Their work would eventually be known collectively as the study of large cardinals.[61]
In 1940, Kurt Gödel showed that the Continuum Hypothesis (CH) cannot be disproved from the axioms of ZFC by showing that both CH and AC hold in his constructible universe: an inner model of ZFC. The existence of a model of ZFC in which additional axioms hold shows that the additional axioms are (relatively) consistent with ZFC.[62] In 1963, Paul Cohen showed that CH cannot be proven from the ZFC axioms, which showed that CH was independent from ZFC. To prove his result, Cohen developed the method of forcing, which has become a standard tool in set theory. Cohen was awarded the Fields Medal in 1966 for his proof.[63][64]
Remove ads
Comparing sets
Summarize
Perspective
Introduction

The basic notions of sets and functions are used to develop the concept of cardinality, and technical terms therein are used throughout this article. A set can be understood as any collection of objects, usually represented with curly braces. For example, specifies a set, called , which contains the numbers 1, 2, and 3. The symbol represents set membership, for example says "1 is a member of the set " which is true by the definition of above.
A function is an association that maps elements of one set to the elements of another, often represented with an arrow diagram. For example, the adjacent image depicts a function which maps the set of natural numbers to the set of even numbers by multiplying by 2. If a function does not map two elements to the same place, it is called injective. If a function covers every element in the output space, it is called surjective. If a function is both injective and surjective, it is called bijective. (For further clarification, see Bijection, injection and surjection.)
Equinumerosity
The intuitive property of two sets having the "same size" is that their objects can be paired one-to-one. A one-to-one pairing between two sets defines a bijective function between them by mapping each object to its pair. Similarly, a bijection between two sets defines a pairing of their elements by pairing each object with the one it maps to. Therefore, these notions of "pairing" and "bijection" are intuitively equivalent.[65] In fact, it is often the case that functions are defined literally as this set of pairings (see Function (mathematics) § Formal definition).[66] Thus, the following definition is given:
Two sets and are said to have the same cardinality if their elements can be paired one-to-one. That is, if there exists a function which is bijective. This is written as and eventually once has been defined.[76] Alternatively, these sets, and may be said to be equivalent, similar, equinumerous, equipotent, or equipollent.[81] For example, the set of non-negative even numbers has the same cardinality as the set of natural numbers, since the function is a bijection from to (see picture above).
The intuitive property for finite sets that "the whole is greater than the part" is no longer true for infinite sets, and the existence of surjections or injections that don't work does not prove that there is no bijection. For example, the function from to , defined by is injective, but not surjective (since 2, for instance, is not mapped to), and from to , defined by (see: floor function) is surjective, but not injective, (since 0 and 1 for instance both map to 0). Neither nor can challenge which was established by the existence of .[82]
Equivalence

A fundamental result necessary in developing a theory of cardinality is relating it to an equivalence relation. A binary relation is an equivalence relation if it satisfies the three basic properties of equality: reflexivity, symmetry, and transitivity.[83]
- Reflexivity: For any set ,
- Given any set there is a bijection from to itself by the identity function, therefore equinumerosity is reflexive.[83]
- Symmetry: If then
- Given any sets and such that there is a bijection from to then there is an inverse function from to which is also bijective, therefore equinumerosity is symmetric.[83]
- Transitivity: If and then
- Given any sets and such that there is a bijection from to and from to then their composition is a bijection from to and so cardinality is transitive.[83]
Since equinumerosity satisfies these three properties, it forms an equivalence relation. This means that cardinality, in some sense, partitions sets into equivalence classes, and one may assign a representative to denote this class. This motivates the notion of a cardinal number.[84] Somewhat more formally, a relation must be a certain set of ordered pairs. Since there is no set of all sets in standard set theory, equinumerosity is not a relation in the usual sense, but a predicate or a relation over classes.
Inequality
A set is not larger than a set if it can be mapped into without overlap. That is, the cardinality of is less than or equal to the cardinality of if there is an injective function from to . This is written and eventually [92] and read as is not greater than or is dominated by [93] If but there is no injection from to then is said to be strictly smaller than written without the underline as or [94] For example, if has four elements and has five, then the following are true and
The basic properties of an inequality are reflexivity (for any ), transitivity (if and then ) and antisymmetry (if and then ) (See Inequality § Formal definitions). Cardinal inequality as defined above is reflexive since the identity function is injective, and is transitive by function composition.[95] Antisymmetry is established by the Schröder–Bernstein theorem. The proof roughly goes as follows.[96]
Given sets and , where is the function that proves and proves , then consider the sequence of points given by applying then to each element over and over. Then we can define a bijection as follows: If a sequence forms a cycle, begins with an element not mapped to by , or extends infinitely in both directions, define for each in those sequences. The last case, if a sequence begins with an element , not mapped to by , define for each in that sequence. Then is a bijection.[96]
The above shows that cardinal inequality is a partial order. A total order has the additional property that, for any and , either or This can be established by the well-ordering theorem. Every well-ordered set is isomorphic to a unique ordinal number, called the order type of the set. Then, by comparing their order types, one can show that or . This result is equivalent to the axiom of choice.[97][98][99]
Remove ads
Countability
Summarize
Perspective
Countable sets
A set is called countable if it is finite or has a bijection with the set of natural numbers in which case it is called countably infinite. The term denumerable is also sometimes used for countably infinite sets.[100] For example, the set of all even natural numbers is countable, and therefore has the same cardinality as the whole set of natural numbers, even though it is a proper subset. Similarly, the set of square numbers is countable, which was considered paradoxical for hundreds of years before modern set theory (cf. § Pre-Cantorian set theory). However, several other examples have historically been considered surprising or initially unintuitive since the rise of set theory.[101]
Two images of a visual depiction of a function from to On the left, a version for the positive rational numbers. On the right, a spiral for all pairs of integers for each fraction
The rational numbers are those which can be expressed as the quotient or fraction of two integers. The rational numbers can be shown to be countable by considering the set of fractions as the set of all ordered pairs of integers, denoted which can be visualized as the set of all integer points on a grid. Then, an intuitive function can be described by drawing a line in a repeating pattern, or spiral, which eventually goes through each point in the grid. For example, going through each diagonal on the grid for positive fractions, or through a lattice spiral for all integer pairs.[102] These technically over cover the rationals, since, for example, the rational number gets mapped to by all the fractions as the grid method treats these all as distinct ordered pairs. So this function shows not This can be corrected by "skipping over" these numbers in the grid, using the Schröder–Bernstein theorem, or by designing a function which does this naturally, for example using the Calkin–Wilf tree.[103]

A number is called algebraic if it is a solution of some polynomial equation (with integer coefficients). For example, the square root of two is a solution to and the rational number is the solution to Conversely, a number which cannot be the root of any polynomial is called transcendental. Two examples include Euler's number (e) and pi (π). In general, proving a number is transcendental is considered to be very difficult, and only a few classes of transcendental numbers are known. However, it can be shown that the set of algebraic numbers is countable by ordering the polynomials lexicographically (for example, see Cantor's first set theory article § The proofs). Since the set of algebraic numbers is countable while the real numbers are uncountable (shown in the following section), the transcendental numbers must form the vast majority of real numbers, even though they are individually much harder to identify. That is to say, almost all real numbers are transcendental.[104]
Hilbert's hotel

Hilbert's paradox of the Grand Hotel is a popular thought experiment devised by the German mathematician David Hilbert to illustrate a counterintuitive property of countably infinite sets, allowing them to have the same cardinality as a proper subset of themselves. The scenario begins by imagining a hotel with an infinite number of rooms, one for each natural number, all of which are occupied. But then a new guest walks in asking for a room. The hotel accommodates by moving the occupant of room 1 to room 2, the occupant of room 2 to room 3, room 3 to room 4, and in general, room n to room n+1. Then every guest still has a room, but room 1 is open for the new guest.[105][106]
Then, the scenario continues by imagining an infinitely long bus of new guests seeking a room. The hotel accommodates by moving the person in room 1 to room 2, room 2 to room 4, and in general, room n to room 2n. Thus, all the even-numbered rooms are occupied, but all the odd-numbered rooms are vacant, leaving room for the infinite bus of new guests. The scenario continues further by assuming an infinite number of these infinite buses arrive at the hotel, and showing that the hotel is still able to accommodate. Finally, an infinite bus which has a seat for every real number arrives, and the hotel is no longer able to accommodate.[105][106]
Uncountable sets
A set is called uncountable if it is not countable; that is, it is infinite and strictly larger than the set of natural numbers. The usual first example of this is the set of real numbers , which can be understood as the set of all numbers on the number line. One method of proving that the reals are uncountable is called Cantor's diagonal argument, credited to Cantor for his 1891 proof,[107] though his method differs from the more common presentation.[108]
It begins by assuming, by contradiction, that there is some one-to-one mapping between the natural numbers and the set of real numbers between 0 and 1 (the interval ). Then, take the decimal representation of each real number, for example, with a leading zero followed by any sequence of digits. The number 1 is included in this set since 1 = 0.999... Considering these real numbers in a column, it is always possible to create a new number such that the first digit of the new number is different from that of the first number in the column, the second digit is different from the second number in the column, and so on. The new number must also have a unique decimal representation, that is, it cannot end in repeating nines or repeating zeros. For example, if the digit isn't 2, make the digit of the new number 2, and if it was 2, make it 3.[109][110] Then, this new number will be different from each of the numbers in the list by at least one digit, and therefore must not be in the list. This shows that the real numbers cannot be put into a one-to-one correspondence with the naturals, and thus must be strictly larger.[111][112]

Another classical example of an uncountable set, established using a related reasoning, is the power set of the natural numbers, denoted . This is the set of all subsets of , including the empty set and itself. The method is much closer to Cantor's original diagonal argument. Again, assume by contradiction that there exists a one-to-one correspondence between and , so that every subset of is assigned to some natural number. These subsets are then placed in a column, in the order defined by (see image). Now, one may define a subset of which is not in the list by taking the negation of the "diagonal" of this column as follows:[113]
If , then , that is, if 1 is in the first subset of the list, then 1 is not in the subset . Further, if , then , that is if the number 2 is not in the second subset of the column, then 2 is in the subset . Then in general, for each natural number , if and only if , meaning is put in the subset only if the nth subset in the column does not contain the number . Then, for each natural number , , meaning, is not the nth subset in the list, for any number , and so it cannot appear anywhere in the list defined by . Since was chosen arbitrarily, this shows that every function from to must be missing at least one element, therefore no such bijection can exist, and so must be not be countable.[113]
These two sets, and can be shown to have the same cardinality (by, for example, assigning each subset to a decimal expansion) called the cardinality of the continuum.[114] Whether there exists a set with cardinality between these two sets is known as the continuum hypothesis.[115]
Cantor's theorem generalizes the second theorem above, showing that every set is strictly smaller than its powerset. The proof roughly goes as follows: Given a set , assume by contradiction that there is a bijection from to . Then, the subset given by taking the negation of the "diagonal", formally, , cannot be in the list. Therefore, every function is missing at least one element, and so . Further, since is itself a set, the argument can be repeated to show . Taking , this shows that is even larger than , which was already shown to be uncountable. Repeating this argument shows that there are infinitely many "sizes" of infinity.[116]
Remove ads
Cardinal numbers
Summarize
Perspective
In the above sections, "the cardinality of a set" was described relationally. In other words, one set could be compared to another, intuitively comparing their "size". Cardinal numbers are a means of measuring this "size" more explicitly. For finite sets, this is simply the natural number found by counting the elements. This number is called the cardinal number of that set, or simply the cardinality of that set. The cardinal number of a set is generally denoted by with a vertical bar on each side,[117] though it may also be denoted by , or [129]
For infinite sets, "cardinal number" is somewhat more difficult to define formally. Cardinal numbers are not usually thought of in terms of their formal definition, but immaterially in terms of their arithmetic/algebraic properties.[130] The assumption that there is some cardinal function which satisfies , sometimes called the axiom of cardinality[131] or Hume's principle,[132] is sufficient for deriving most properties of cardinal numbers.[133]
Commonly in mathematics, if a relation satisfies the properties of an equivalence relation, the objects used to materialize this relation are equivalence classes, which groups all the objects equivalent to one another. These called the Frege–Russell cardinal numbers.[134] However, this would mean that cardinal numbers are too large to form sets (apart from the cardinal number whose only element is the empty set), since, for example, the cardinal number would be the set of all sets with one element, and would therefore be a proper class.[135] Thus, due to John von Neumann, it is more common to assign representatives of these classes.[136]
Finite sets

Given a basic sense of natural numbers, a set is said to have cardinality if it can be put in one-to-one correspondence with the set analogous to counting its elements.[137][138] For example, the set has a natural correspondence with the set and therefore is said to have cardinality 4. Other terminologies include "Its cardinality is 4" or "Its cardinal number is 4". In formal contexts, the natural numbers can be understood as some construction of objects satisfying the Peano axioms.[139]
Showing that such a correspondence exists is not always trivial. Combinatorics is the area of mathematics primarily concerned with counting, both as a means and as an end to obtaining results, and certain properties of finite structures.[140] The notion cardinality of finite sets is closely tied to many basic combinatorial principles, and provides a set-theoretic foundation to prove them. It can be shown by induction on the possible sizes of sets that finite cardinality corresponds uniquely with natural numbers (cf. Finite set § Uniqueness of cardinality).[141] This is related—though not equivalent—to the pigeonhole principle.[142]
The addition principle asserts that given disjoint sets and , , intuitively meaning that the sum of the parts is equal to the whole.[143] The multiplication principle asserts that given two sets and , , intuitively meaning that there are ways to pair objects from these sets.[144] Both of these can be proven by a bijective proof, together with induction.[145] The more general result is the inclusion–exclusion principle, which defines how to count the number of elements in overlapping sets.[146]
Naturally, a set is defined to be finite if it is empty or can be put in correspondence with the set for some natural number [137][138] However, there exist other definitions of "finite" which don't rely on a definition of "number." For example, a set is called Dedekind-finite if it cannot be put in one-to-one correspondece with a proper subset of itself.[147]
Aleph numbers
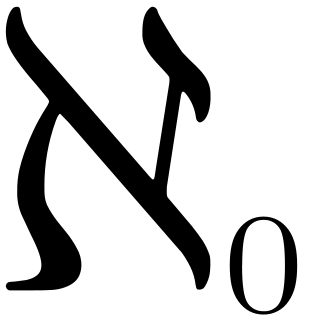
The aleph numbers are a sequence of cardinal numbers that represent the sizes of infinite sets, denoted with an aleph the first letter of the Hebrew alphabet.[148] The first aleph number is called "aleph-nought", "aleph-zero", or "aleph-null", which represents the cardinality of the set of all natural numbers: Then, represents the next largest cardinality, then , and so on.[149] The most common way this is formalized in set theory is through Von Neumann ordinals, known as Von Neumann cardinal assignment.[150]
Ordinal numbers generalize the notion of order to infinite sets. For example, 2 comes after 1, denoted and 3 comes after both, denoted Then, one defines a new number, which comes after every natural number, denoted Further and so on.[151] More formally, these ordinal numbers can be defined as follows:
the empty set, and so on. Then one can define for example, therefore Defining (a limit ordinal) gives the desired property of being the smallest ordinal greater than all finite ordinal numbers. Further, , and so on.[152]
Since by the natural correspondence, one may define as the set of all finite ordinals. That is, Then, is the set of all countable ordinals (all ordinals with cardinality ), the first uncountable ordinal. Since a set cannot contain itself, must have a strictly larger cardinality: [153] Furthermore, is the set of all ordinals with cardinality less than or equal to and in general the successor cardinal is the set of all ordinals with cardinality up to . Put another way for infinite cardinals, is the number of possible well-orderings on up to order isomorphism.[154] This is sometimes called the Hartogs number of .[155] Then, for a limit ordinal is the union of all lesser alephs.[156] By the well-ordering theorem, there cannot exist any set with cardinality between and and every infinite set has some cardinality corresponding to some aleph for some ordinal [157]
Cardinal arithmetic

Basic arithmetic can be done on cardinal numbers in a very natural way, by extending the theorems for finite combinatorial principles above. The intuitive principle that is and are disjoint then addition of these sets is simply taking their union, written as . Thus if and are infinite, cardinal addition is defined as where denotes disjoint union. Similarly, the multiplication of two sets is intuitively the number of ways to pair their elements (as in the multiplication principle), therefore cardinal multiplication is defined as , where denotes the Cartesian product. These definitions can be shown to satisfy the basic properties of standard arithmetic:
- Associativity: , and
- Commutativity: , and
- Distributivity:
Cardinal exponentiation is defined via set exponentiation, the set of all functions , that is, For finite sets this can be shown to coincide with standard natural number exponentiation, but includes as a corollary that zero to the power of zero is one since there is exactly one function from the empty set to itself: the empty function. A combinatorial argument can be used to show . In general, cardinal exponentiation is not as well-behaved as cardinal addition and multiplication. For example, even though it can be proven that the expression does indeed correspond to some aleph, it is unprovable from standard set theories which aleph it corresponds to.
Set of all cardinal numbers
The set of all cardinal numbers refers to a hypothetical set which contains all cardinal numbers. Such a set cannot exist, which has been considered paradoxical, and related to the Burali-Forti paradox. Using the definition of cardinal numbers as representatives of their cardinalities. It begins by assuming there is some set Then, if there is some largest cardinal then the powerset is strictly greater, and thus not in Conversely, if there is no largest element, then the union contains the elements of all elements of and is therefore greater than or equal to each element. Since there is no largest element in for any element there is another element such that and Thus, for any and so Thus, the collection of cardinal numbers is too large to form a set, and is a proper class.
Remove ads
Cardinality of the continuum

The number line is a geometric construct of the intuitive notions of "space" and "distance" wherein each point corresponds to a distinct quantity or position along a continuous path. The terms "continuum" and "continuous" refer to the totality of this line, having some space (other points) between any two points on the line (dense and archimedian) and the absence of any gaps (completeness), This intuitive construct is formalized by the set of real numbers which model the continuum as a complete, densely ordered, uncountable set.

The cardinality of the continuum, denoted by "" (a lowercase fraktur script "c"), remains invariant under various transformations and mappings, many considered surprising. For example, all intervals on the real line e.g. , and , have the same cardinality as the entire set . First, is a bijection from to . Then, the tangent function is a bijection from the interval to the whole real line. A more surprising example is the Cantor set, which is defined as follows: take the interval and remove the middle third , then remove the middle third of each of the two remaining segments, and continue removing middle thirds (see image). The Cantor set is the set of points that survive this process. This set that remains is all of the points whose decimal expansion can be written in ternary without a 1. Reinterpreting these decimal expansions as binary (e.g. by replacing the 2s with 1s) gives a bijection between the Cantor set and the interval .

Space-filling curves are continuous surjective maps from the unit interval onto the unit square on , with classical examples such as the Peano curve and Hilbert curve. Although such maps are not injective, they are indeed surjective, and thus suffice to demonstrate cardinal equivalence. They can be reused at each dimension to show that for any dimension The infinite cartesian product , can also be shown to have cardinality . This can be established by cardinal exponentiation: . Thus, the real numbers, all finite-dimensional real spaces, and the countable cartesian product share the same cardinality.
As shown in § Uncountable sets, the set of real numbers is strictly larger than the set of natural numbers. Specifically, . The Continuum Hypothesis (CH) asserts that the real numbers have the next largest cardinality after the natural numbers, that is . As shown by Gödel and Cohen, the continuum hypothesis is independent of ZFC, a standard axiomatization of set theory; that is, it is impossible to prove the continuum hypothesis or its negation from ZFC—provided that ZFC is consistent.[158][159][160] The Generalized Continuum Hypothesis (GCH) extends this to all infinite cardinals, stating that for every ordinal . Research on CH and GCH continues independent of ZFC, especially in descriptive set theory and through the exploration of large cardinal axioms.[161] Without GHC, the cardinality of cannot be written in terms of specific alephs. The Beth numbers provide a concise notation for powersets of the real numbers starting from , then , and , and in general and if is a limit ordinal.
Remove ads
Skolem's paradox

In model theory, a model corresponds to a specific interpretation of a formal language or theory. It consists of a domain (a set of objects) and an interpretation of the symbols in the language, such that the axioms of the theory are satisfied within this structure. In first-order logic, the Löwenheim–Skolem theorem states that if a theory has an infinite model, then it also has models of every other infinite cardinality. Applied to set theory, it asserts that Zermelo–Fraenkel set theory, which proves the existence of uncountable sets such as , nevertheless has a countable model. Thus, Skolem's paradox was posed as follows: how can it be that there exists a domain of set theory which only contains countably many objects, but is capable of satisfying the statement "there exists a set with uncountably many elements"?[162]
Skolem's paradox does not only apply to ZFC, but any first-order set theory, if it is consistent, has a model which is countable. A mathematical explanation of the paradox, showing that it is not a true contradiction in mathematics, was first given in 1922 by Thoralf Skolem. He explained that the countability or uncountability of a set is not absolute, but relative to the model in which the cardinality is measured. This is because, for example, if the set is countable in a model of set theory then there is a bijection But a submodel containing which excludes all such functions would thus contain no bijection between and , and therefore would be uncountable. In second-order and higher-order logics, the Löwenheim–Skolem theorem does not hold. This is due to the fact that second-order logic quantifies over all subsets of the domain. Skolem's work was harshly received by Ernst Zermelo, who argued against the limitations of first-order logic and Skolem's notion of "relativity", but the result quickly came to be accepted by the mathematical community.[163][162]
Remove ads
Alternative and additional axioms
Summarize
Perspective
Around the turn of the 20th century, set theory turned to an axiomatic approach to avoid rampant foundational issues related to its naive study (cf. § Axiomatic set theory). The most common axiomatic set theory used today is Zermelo–Fraenkel set theory (ZFC).[60] In this system, the relevant axioms include: the Axiom of Infinity, stating roughly "there is an infinite set", specifically, a set with cardinality of the natural numbers ; the Axiom of power set, which says that, for any set , the powerset also exists; and the Axiom of Choice, explained below. ZFC has been criticized both for being too strong, and too weak. Similarly, there are many "natural extensions" of ZFC studied by set theorists. Thus, there exist many alternative systems of axioms each of which has implications to the standard theory of cardinality discussed above.
Without the axiom of choice
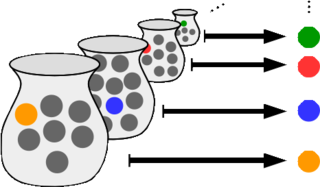
The Axiom of Choice (AC) is a fundamental principle in the foundations of mathematics which has seen much controversy in its history. Roughly, it states that given any collection of non-empty sets, it is possible to construct a new set by choosing one element from each set, even if the collection is infinite. In many cases, a set created by choosing elements can be made without invoking the axiom of choice. But it is not possible to do this in general, in which case, the axiom of choice must be invoked.
If the Axiom of Choice is assumed to be false in ZF, it has several implications:
- Cardinal inequality cannot be a total order on all sets. That is, given any two sets it may be that neither nor hold. Further, since Alephs can naturally be compared, there exist sets which do not correspond to any Aleph number.
- There may exist sets which are Dedekind-finite, meaning they cannot be put in bijection with a proper subset of itself, but whose elements cannot be counted (put in bijection with for any n).
- It is impossible to prove there exists a cardinal function which satisfies the cardinal number axiom, and satisfies for all [164] Thus, some authors reserve for the cardinality of well-orderable sets. Still, the function can be defined using Scott's trick, which uses the Axiom of Regularity to assign each set to the least rank of the Von Neumann universe which contains a set equinumerous to
- One may define the "infinite product" of cardinal numbers as the cardinality of the set of all sequences of their elements. Asserting that this set is always nonempty is equivalent to the axiom of choice. This is why Bertrand Russell called AC the Multiplicative axiom.
- Although the Generalized Continuum Hypothesis (GCH) is independent of ZFC, it can be shown that ZF+GCH is sufficient to prove AC. Thus, if AC is false, it follows that the GCH does not hold.
Proper classes

Some set theories allow for proper classes, which are, roughly, collections "too large" to form sets. For example, the Universe of all sets, the class of all cardinal numbers, or the class of all ordinal numbers. Such set theories include Von Neumann–Bernays–Gödel set theory, and Morse–Kelley set theory. In such set theories, some authors find this definition more elegant than assigning representatives, as it more accurately describes the concept by "definition by abstraction".
Proper classes can, in a sense, be assigned cardinalities. Cantor originally called the cardinality of a proper class "Absolute infinite", denoted with tav ת (the last letter of the Hebrew alphabet), and associated the concept with God.[165] The first to distinguish between sets and classes was John von Neumann, who formalized the notion of "too large to form sets". More accurately, he defined a class to be a proper class if and only if it was equinumerous with the whole Universe of sets (cf. Axiom of limitation of size). Thus, all proper classes have the same "size". The axiom has several implications, mostly relating to the limitation of size principles of early set theory. It implies the axiom of specification, the axiom of replacement, the axiom of union, and the axiom of global choice.
Large cardinals
Large cardial axioms assert the existence of cardinal numbers which, as the name suggests, are very large—so large that they cannot be proven to exist within ZFC. For example, an inaccessible cardinal is, roughly, a cardinal that cannot be approached from below using basic set-theoretic operations such as unions, limits, and powersets (more formally, a regular, limit cardinal). Large cardinals are understood in terms of the Von Neumann hierarchy, denoted (for some ordinal ), which can be roughly understood as the sets that can be obtained from the empty set, followed by recursively applying the powerset times. Specifically, , and for a limit ordinal .[166]
There exist many known properties defining large cardinals, which come roughly in a linear higherarchy, in terms of consistency strength. As an analogy, in ZFC without the Axiom of Infinity can only prove the existence of finite sets. Therefore , whose existence is provable in usual ZFC, can serve as a model of ZFC–Infinity, and thus if ZFC is consistent, ZFC–Infinity is consistent.[167] Analogously, ZFC+"There exists an inaccessible cardinal" implies the consistency of ZFC, since if is inaccessible, can serve as a model of ZFC (cf. Grothendieck universe). Stronger and stronger large cardinal axioms assert the existence of larger and larger cardinals, each of which proves the consistency of the weaker systems.[168]
Large cardinals are at the forefront of set-theoretic research for both practical and philosophical reasons. In a practical sense, it is often the case that unproven or unprovable conjectures can be settled by sufficiently strong large cardinal axioms. In a philosophical sense, accoring to a platonic view among set-theorists such as W. Hugh Woodin, these axioms simply extend the system to include sets that are "supposed" to be considered. That is, there is some fundamental universe of sets, which these axioms grant further access to.[169] For this reason, large cardinal axioms are generally given preference compared to other possible axioms of set theory. This view is controversial among a competing philosophy, sometimes called pluralsim,[170] which posits that set theory should be understood as a multiverse of set theories, but no "absolute" or "true" model.[171]
Constructability
The axiom of constructibility was the axiom introduced by Kurt Gödel to prove the relative consistency of the axiom of choice and generalized continuum hypothesis. It states that all sets are "constructable" (cf. Constructible universe § What L is). It has several consequences, naturally, including AC and GCH, but resolves several other important questions in set theory. The axiom, however, is not well-accepted by set-theorists, most believing it to be "too restrictive". In part it is because the axiom is contradicted by sufficiently strong large cardinal axioms.
Determinacy

The Axiom of Determinacy (AD) asserts that certain kinds of mathematical games on the natural numbers are determined; that is, one player will always have a guaranteed winning strategy.[172] The first serious study of the consiquences of AD started durring the 1960s in descriptive set theory—which, roughly, studies the definable sets of the real numbers—after it was noticed that it leads to very nice regulatory properties of the real numbers.[173] Specifically, it implies the perfect set property, the property of Baire, and that all sets of real numbers are Lebesgue measurable.[174] However, it was shown that this axiom is inconsistent with AC (cf. Axiom of determinacy § Incompatibility with the axiom of choice), and thus was never taken as a fundamental axiom of set theory.[175] However, its relationship to and consistency with large cardinals is still of heavy interest among set theorists such as Donald A. Martin, John R. Steel, and W. Hugh Woodin.[176]
Replacing the Axiom of Choice with the Axiom of Determinacy has several implications:
- The Banach–Tarski paradox is a paradox arising from AC which allows one to disassemble a ball into finitely many pieces, and re-arrange them into two balls identical to the original. This, and related paradoxes, become impossible under AD.[177]
Remove ads
See also
Wikimedia Commons has media related to Cardinality.
Wikidata has the properties:
References
Wikiwand - on
Seamless Wikipedia browsing. On steroids.
Remove ads












![{\displaystyle [0,5]}](http://wikimedia.org/api/rest_v1/media/math/render/svg/466c74a63ec8b8422ccb96c6bc5140dca45b5593)
![{\displaystyle [0,12]}](http://wikimedia.org/api/rest_v1/media/math/render/svg/89aabd85b3e389da4856956942ab5320c9bc586f)






![{\displaystyle [0,1]}](http://wikimedia.org/api/rest_v1/media/math/render/svg/738f7d23bb2d9642bab520020873cccbef49768d)






































































































































































 ,
,  ...
...























![{\displaystyle [0,2]}](http://wikimedia.org/api/rest_v1/media/math/render/svg/120ef5837b0c64a40a2333f5aefd3c36fc458e91)































