In geometry, the quarter hypercubic honeycomb (or quarter n-cubic honeycomb) is a dimensional infinite series of honeycombs, based on the hypercube honeycomb. It is given a Schläfli symbol q{4,3...3,4} or Coxeter symbol qδ4 representing the regular form with three quarters of the vertices removed and containing the symmetry of Coxeter group 
for n ≥ 5, with 
= 
and for quarter n-cubic honeycombs 
= 
.[1]
More information qδn, Name ...
| qδn |
Name |
Schläfli
symbol |
Coxeter diagrams |
Facets |
Vertex figure |
|---|
| qδ3 |
quarter square tiling |
q{4,4} |
   or 
 or 
  
|
h{4}={2} |
{ }×{ } |
|
{ }×{ } |
| qδ4 |
quarter cubic honeycomb |
q{4,3,4} |
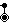
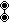   or  
  or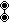
 |
h{4,3} |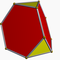 
h2{4,3} |
|
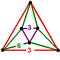
Elongated
triangular antiprism |
| qδ5 |
quarter tesseractic honeycomb |
q{4,32,4} |
    or  
or
|
h{4,32} | 
h3{4,32} |
|
{3,4}×{} |
| qδ6 |
quarter 5-cubic honeycomb |
q{4,33,4} |
|
h{4,33} | 
h4{4,33} |
|
Rectified 5-cell antiprism |
| qδ7 |
quarter 6-cubic honeycomb |
q{4,34,4} |
|
h{4,34} | 
h5{4,34} |
{3,3}×{3,3} |
| qδ8 |
quarter 7-cubic honeycomb |
q{4,35,4} |
|
h{4,35} | 
h6{4,35} |
{3,3}×{3,31,1} |
| qδ9 |
quarter 8-cubic honeycomb |
q{4,36,4} |
|
h{4,36} | 
h7{4,36} |
{3,3}×{3,32,1}
{3,31,1}×{3,31,1} |
| |
| qδn |
quarter n-cubic honeycomb |
q{4,3n−3,4} |
... |
h{4,3n−2} |
hn−2{4,3n−2} |
... |
Close
