Top Qs
Timeline
Chat
Perspective
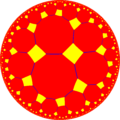
Order-4 hexagonal tiling
Regular tiling of the hyperbolic plane From Wikipedia, the free encyclopedia
Remove ads
In geometry, the order-4 hexagonal tiling is a regular tiling of the hyperbolic plane. It has Schläfli symbol of {6,4}.
| Order-4 hexagonal tiling | |
|---|---|
 Poincaré disk model of the hyperbolic plane | |
| Type | Hyperbolic regular tiling |
| Vertex configuration | 64 |
| Schläfli symbol | {6,4} |
| Wythoff symbol | 4 | 6 2 |
| Coxeter diagram | |
| Symmetry group | [6,4], (*642) |
| Dual | Order-6 square tiling |
| Properties | Vertex-transitive, edge-transitive, face-transitive |
Remove ads
Symmetry
This tiling represents a hyperbolic kaleidoscope of 6 mirrors defining a regular hexagon fundamental domain. This symmetry by orbifold notation is called *222222 with 6 order-2 mirror intersections. In Coxeter notation can be represented as [6*,4], removing two of three mirrors (passing through the hexagon center). Adding a bisecting mirror through 2 vertices of a hexagonal fundamental domain defines a trapezohedral *4422 symmetry. Adding 3 bisecting mirrors through the vertices defines *443 symmetry. Adding 3 bisecting mirrors through the edge defines *3222 symmetry. Adding all 6 bisectors leads to full *642 symmetry.
 *222222 |
 *443 |
 *3222 |
 *642 |
Remove ads
Uniform colorings
There are 7 distinct uniform colorings for the order-4 hexagonal tiling. They are similar to 7 of the uniform colorings of the square tiling, but exclude 2 cases with order-2 gyrational symmetry. Four of them have reflective constructions and Coxeter diagrams while three of them are undercolorings.
Remove ads
Regular maps
The regular map {6,4}3 or {6,4}(4,0) can be seen as a 4-coloring on the {6,4} tiling. It also has a representation as a petrial octahedron, {3,4}π, an abstract polyhedron with vertices and edges of an octahedron, but instead connected by 4 Petrie polygon faces.
Related polyhedra and tiling
Summarize
Perspective
This tiling is topologically related as a part of sequence of regular tilings with hexagonal faces, starting with the hexagonal tiling, with Schläfli symbol {6,n}, and Coxeter diagram ![]()
![]()
![]()
![]()
![]() , progressing to infinity.
, progressing to infinity.
This tiling is also topologically related as a part of sequence of regular polyhedra and tilings with four faces per vertex, starting with the octahedron, with Schläfli symbol {n,4}, and Coxeter diagram ![]()
![]()
![]()
![]()
![]() , with n progressing to infinity.
, with n progressing to infinity.
| Uniform tilings in symmetry *3222 | ||||
|---|---|---|---|---|
 |
 |
 |
 | |
 |
 |
 | ||
 |
 |
 | ||
Remove ads
See also
Wikimedia Commons has media related to Order-4 hexagonal tiling.
References
- John H. Conway, Heidi Burgiel, Chaim Goodman-Strauss, The Symmetries of Things 2008, ISBN 978-1-56881-220-5 (Chapter 19, The Hyperbolic Archimedean Tessellations)
- "Chapter 10: Regular honeycombs in hyperbolic space". The Beauty of Geometry: Twelve Essays. Dover Publications. 1999. ISBN 0-486-40919-8. LCCN 99035678.
External links
Wikiwand - on
Seamless Wikipedia browsing. On steroids.
Remove ads